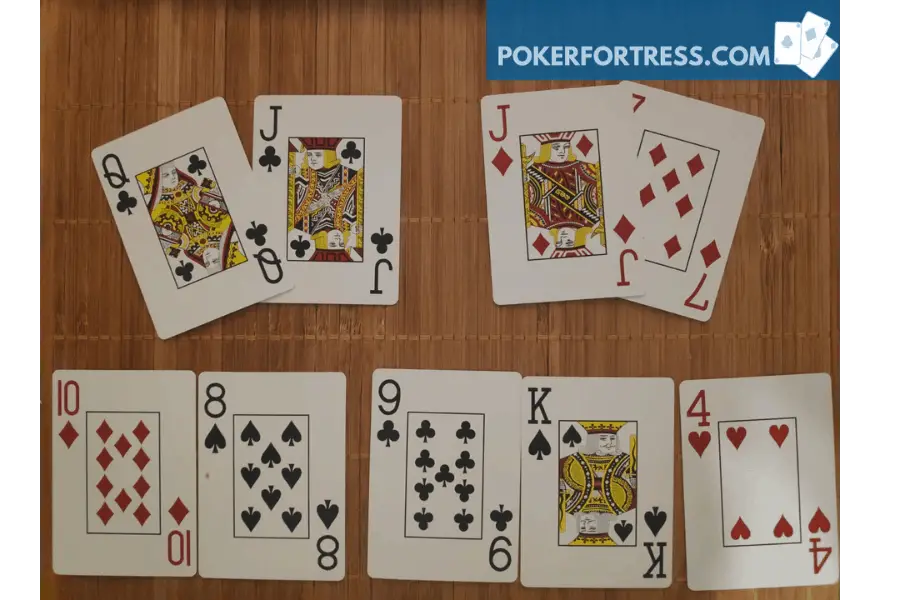
A Basic Calling Scenario In a $0.05/0.10 game with $2.25 stacks before the blinds are posted, it folds to the small blind who goes all-in. We're in the big blind and decide to call. Members can review and practice LearnWPT Poker Hand scenarios all in one place. Hand analysis is one of the best things you can do away from the table to improve your results at the table and with a LearnWPT Membership you can practice anytime! LearnWPT is a Membership based poker learning site dedicated to providing education and instruction of functional strategies built to improve the games.
This post works with 5-card Poker hands drawn from a standard deck of 52 cards. The discussion is mostly mathematical, using the Poker hands to illustrate counting techniques and calculation of probabilities
Working with poker hands is an excellent way to illustrate the counting techniques covered previously in this blog – multiplication principle, permutation and combination (also covered here). There are 2,598,960 many possible 5-card Poker hands. Thus the probability of obtaining any one specific hand is 1 in 2,598,960 (roughly 1 in 2.6 million). The probability of obtaining a given type of hands (e.g. three of a kind) is the number of possible hands for that type over 2,598,960. Thus this is primarily a counting exercise.
___________________________________________________________________________
Preliminary Calculation
Usually the order in which the cards are dealt is not important (except in the case of stud poker). Thus the following three examples point to the same poker hand. The only difference is the order in which the cards are dealt.
These are the same hand. Order is not important.
The number of possible 5-card poker hands would then be the same as the number of 5-element subsets of 52 objects. The following is the total number of 5-card poker hands drawn from a standard deck of 52 cards.
The notation is called the binomial coefficient and is pronounced 'n choose r', which is identical to the number of -element subsets of a set with objects. Other notations for are , and . Many calculators have a function for . Of course the calculation can also be done by definition by first calculating factorials.

Thus the probability of obtaining a specific hand (say, 2, 6, 10, K, A, all diamond) would be 1 in 2,598,960. If 5 cards are randomly drawn, what is the probability of getting a 5-card hand consisting of all diamond cards? It is
This is definitely a very rare event (less than 0.05% chance of happening). The numerator 1,287 is the number of hands consisting of all diamond cards, which is obtained by the following calculation.
The reasoning for the above calculation is that to draw a 5-card hand consisting of all diamond, we are drawing 5 cards from the 13 diamond cards and drawing zero cards from the other 39 cards. Since (there is only one way to draw nothing), is the number of hands with all diamonds.
If 5 cards are randomly drawn, what is the probability of getting a 5-card hand consisting of cards in one suit? The probability of getting all 5 cards in another suit (say heart) would also be 1287/2598960. So we have the following derivation.
Thus getting a hand with all cards in one suit is 4 times more likely than getting one with all diamond, but is still a rare event (with about a 0.2% chance of happening). Some of the higher ranked poker hands are in one suit but with additional strict requirements. They will be further discussed below.
Another example. What is the probability of obtaining a hand that has 3 diamonds and 2 hearts? The answer is 22308/2598960 = 0.008583433. The number of '3 diamond, 2 heart' hands is calculated as follows:
One theme that emerges is that the multiplication principle is behind the numerator of a poker hand probability. For example, we can think of the process to get a 5-card hand with 3 diamonds and 2 hearts in three steps. The first is to draw 3 cards from the 13 diamond cards, the second is to draw 2 cards from the 13 heart cards, and the third is to draw zero from the remaining 26 cards. The third step can be omitted since the number of ways of choosing zero is 1. In any case, the number of possible ways to carry out that 2-step (or 3-step) process is to multiply all the possibilities together.
___________________________________________________________________________
The Poker Hands
Here's a ranking chart of the Poker hands.
The chart lists the rankings with an example for each ranking. The examples are a good reminder of the definitions. The highest ranking of them all is the royal flush, which consists of 5 consecutive cards in one suit with the highest card being Ace. There is only one such hand in each suit. Thus the chance for getting a royal flush is 4 in 2,598,960.
Royal flush is a specific example of a straight flush, which consists of 5 consecutive cards in one suit. There are 10 such hands in one suit. So there are 40 hands for straight flush in total. A flush is a hand with 5 cards in the same suit but not in consecutive order (or not in sequence). Thus the requirement for flush is considerably more relaxed than a straight flush. A straight is like a straight flush in that the 5 cards are in sequence but the 5 cards in a straight are not of the same suit. For a more in depth discussion on Poker hands, see the Wikipedia entry on Poker hands.
The counting for some of these hands is done in the next section. The definition of the hands can be inferred from the above chart. For the sake of completeness, the following table lists out the definition.
Definitions of Poker Hands
| Poker Hand | Definition | |
|---|---|---|
| 1 | Royal Flush | A, K, Q, J, 10, all in the same suit |
| 2 | Straight Flush | Five consecutive cards, |
| all in the same suit | ||
| 3 | Four of a Kind | Four cards of the same rank, |
| one card of another rank | ||
| 4 | Full House | Three of a kind with a pair |
| 5 | Flush | Five cards of the same suit, |
| not in consecutive order | ||
| 6 | Straight | Five consecutive cards, |
| not of the same suit | ||
| 7 | Three of a Kind | Three cards of the same rank, |
| 2 cards of two other ranks | ||
| 8 | Two Pair | Two cards of the same rank, |
| two cards of another rank, | ||
| one card of a third rank | ||
| 9 | One Pair | Three cards of the same rank, |
| 3 cards of three other ranks | ||
| 10 | High Card | If no one has any of the above hands, |
| the player with the highest card wins |
___________________________________________________________________________
Counting Poker Hands
Straight Flush
Counting from A-K-Q-J-10, K-Q-J-10-9, Q-J-10-9-8, …, 6-5-4-3-2 to 5-4-3-2-A, there are 10 hands that are in sequence in a given suit. So there are 40 straight flush hands all together.
Four of a Kind
There is only one way to have a four of a kind for a given rank. The fifth card can be any one of the remaining 48 cards. Thus there are 48 possibilities of a four of a kind in one rank. Thus there are 13 x 48 = 624 many four of a kind in total.
Full House
Let's fix two ranks, say 2 and 8. How many ways can we have three of 2 and two of 8? We are choosing 3 cards out of the four 2's and choosing 2 cards out of the four 8's. That would be = 4 x 6 = 24. But the two ranks can be other ranks too. How many ways can we pick two ranks out of 13? That would be 13 x 12 = 156. So the total number of possibilities for Full House is
Note that the multiplication principle is at work here. When we pick two ranks, the number of ways is 13 x 12 = 156. Why did we not use = 78?
Flush
There are = 1,287 possible hands with all cards in the same suit. Recall that there are only 10 straight flush on a given suit. Thus of all the 5-card hands with all cards in a given suit, there are 1,287-10 = 1,277 hands that are not straight flush. Thus the total number of flush hands is 4 x 1277 = 5,108.
Straight
There are 10 five-consecutive sequences in 13 cards (as shown in the explanation for straight flush in this section). In each such sequence, there are 4 choices for each card (one for each suit). Thus the number of 5-card hands with 5 cards in sequence is . Then we need to subtract the number of straight flushes (40) from this number. Thus the number of straight is 10240 – 10 = 10,200.
Three of a Kind
There are 13 ranks (from A, K, …, to 2). We choose one of them to have 3 cards in that rank and two other ranks to have one card in each of those ranks. The following derivation reflects all the choosing in this process.
Two Pair and One Pair
These two are left as exercises.
High Card
The count is the complement that makes up 2,598,960.
The following table gives the counts of all the poker hands. The probability is the fraction of the 2,598,960 hands that meet the requirement of the type of hands in question. Note that royal flush is not listed. This is because it is included in the count for straight flush. Royal flush is omitted so that he counts add up to 2,598,960.
Probabilities of Poker Hands
| Poker Hand | Count | Probability | |
|---|---|---|---|
| 2 | Straight Flush | 40 | 0.0000154 |
| 3 | Four of a Kind | 624 | 0.0002401 |
| 4 | Full House | 3,744 | 0.0014406 |
| 5 | Flush | 5,108 | 0.0019654 |
| 6 | Straight | 10,200 | 0.0039246 |
| 7 | Three of a Kind | 54,912 | 0.0211285 |
| 8 | Two Pair | 123,552 | 0.0475390 |
| 9 | One Pair | 1,098,240 | 0.4225690 |
| 10 | High Card | 1,302,540 | 0.5011774 |
| Total | 2,598,960 | 1.0000000 |
___________________________________________________________________________
2017 – Dan Ma
In last week's article, we looked at the basic components of EV calculations. We made two very important points that we're going to use this week:
- The EV of an event is equal to the EVs of each possible outcome added together.
- The EV of an individual outcome is the chance it happens multiplied by the profit when it happens.
We also broke down how to find the EV of any event into a three-step process. If you aren't familiar with that process, then go back and read last week's article because that's the process that we're going to use to evaluate the expected values of some poker situations this week.
For the time being, we aren't going to be concerned with factoring in the rake for these calculations. Once you learn how to do these calculations on a basic level, it's easy to compensate for the rake, and we'll do just that once we get into more in-depth problems.
A Basic Bluffing Scenario
Suppose we're on the river with the nut low against a single opponent who has us covered. The pot is $15, and we make a bet of $10. If our opponent folds, then we win the hand. If our opponent does anything besides fold, then we lose. What is the EV of our bluff if we estimate that our opponent will fold 35 percent of the time?
The first thing we need to do is identify all of the possible outcomes. In this scenario, there are really only two possible outcomes. Our opponent can fold, or our opponent will not fold. This gives us the following EV equation for the value of our bluff:
EV of our bluff = EV of opponent folding + EV of opponent not folding
Now we need to find the EV of the outcome of our opponent folding. To do this, we need to know our profit when our opponent folds and the chance of our opponent folding. We will profit the $15 pot when our opponent mucks his hand, and it will happen 35 percent of the time. We multiply these values together to get the EV of the outcome.
EV of opponent folding = $15 * 0.35 = $5.25
Next, we have to find the EV of our opponent not folding. This will happen 65 percent of the time, and our profit will be a loss of $10 since we lose our bet.
EV of opponent not folding = -$10 * 0.65 = -$6.50
Finally, we add the EV of each outcome together to get our overall EV of our bluff.
EV of our bluff = EV of opponent folding + EV of opponent not folding
EV of our bluff = $5.25 + (-$6.50) = -$1.25
So on average, we will lose $1.25 each time that we make this bluff.
A Basic Calling Scenario
In a $0.05/0.10 game with $2.25 stacks before the blinds are posted, it folds to the small blind who goes all-in. We're in the big blind and decide to call. If we have 55 percent equity against our opponent's range, then what is the EV of our call?
First thing's first: We have to figure out all of the possible outcomes. For our purposes, we can say that we'll either win or lose. That gives us the following EV equation:
EV of calling = EV of winning + EV of losing
Like before, we have to find the EV of each of these outcomes to find the overall EV of calling. The chance of winning is 55 percent since that's our equity, and we'll profit $2.35 because we'll get our opponent's entire starting stack plus our $0.10 blind that was posted. That makes the EV of the winning outcome the following:
EV of winning = $2.35 * 0.55 = $1.29
Now let's find the EV of losing. We know that we'll lose 45 percent of the time because we have 55 percent equity. When we call, we'll actually be calling $2.15 since our starting stack was $2.25 and we posted a $0.10 blind. That means that if we lose, we're losing $2.15.
Thus the probability of obtaining a specific hand (say, 2, 6, 10, K, A, all diamond) would be 1 in 2,598,960. If 5 cards are randomly drawn, what is the probability of getting a 5-card hand consisting of all diamond cards? It is
This is definitely a very rare event (less than 0.05% chance of happening). The numerator 1,287 is the number of hands consisting of all diamond cards, which is obtained by the following calculation.
The reasoning for the above calculation is that to draw a 5-card hand consisting of all diamond, we are drawing 5 cards from the 13 diamond cards and drawing zero cards from the other 39 cards. Since (there is only one way to draw nothing), is the number of hands with all diamonds.
If 5 cards are randomly drawn, what is the probability of getting a 5-card hand consisting of cards in one suit? The probability of getting all 5 cards in another suit (say heart) would also be 1287/2598960. So we have the following derivation.
Thus getting a hand with all cards in one suit is 4 times more likely than getting one with all diamond, but is still a rare event (with about a 0.2% chance of happening). Some of the higher ranked poker hands are in one suit but with additional strict requirements. They will be further discussed below.
Another example. What is the probability of obtaining a hand that has 3 diamonds and 2 hearts? The answer is 22308/2598960 = 0.008583433. The number of '3 diamond, 2 heart' hands is calculated as follows:
One theme that emerges is that the multiplication principle is behind the numerator of a poker hand probability. For example, we can think of the process to get a 5-card hand with 3 diamonds and 2 hearts in three steps. The first is to draw 3 cards from the 13 diamond cards, the second is to draw 2 cards from the 13 heart cards, and the third is to draw zero from the remaining 26 cards. The third step can be omitted since the number of ways of choosing zero is 1. In any case, the number of possible ways to carry out that 2-step (or 3-step) process is to multiply all the possibilities together.
___________________________________________________________________________
The Poker Hands
Here's a ranking chart of the Poker hands.
The chart lists the rankings with an example for each ranking. The examples are a good reminder of the definitions. The highest ranking of them all is the royal flush, which consists of 5 consecutive cards in one suit with the highest card being Ace. There is only one such hand in each suit. Thus the chance for getting a royal flush is 4 in 2,598,960.
Royal flush is a specific example of a straight flush, which consists of 5 consecutive cards in one suit. There are 10 such hands in one suit. So there are 40 hands for straight flush in total. A flush is a hand with 5 cards in the same suit but not in consecutive order (or not in sequence). Thus the requirement for flush is considerably more relaxed than a straight flush. A straight is like a straight flush in that the 5 cards are in sequence but the 5 cards in a straight are not of the same suit. For a more in depth discussion on Poker hands, see the Wikipedia entry on Poker hands.
The counting for some of these hands is done in the next section. The definition of the hands can be inferred from the above chart. For the sake of completeness, the following table lists out the definition.
Definitions of Poker Hands
| Poker Hand | Definition | |
|---|---|---|
| 1 | Royal Flush | A, K, Q, J, 10, all in the same suit |
| 2 | Straight Flush | Five consecutive cards, |
| all in the same suit | ||
| 3 | Four of a Kind | Four cards of the same rank, |
| one card of another rank | ||
| 4 | Full House | Three of a kind with a pair |
| 5 | Flush | Five cards of the same suit, |
| not in consecutive order | ||
| 6 | Straight | Five consecutive cards, |
| not of the same suit | ||
| 7 | Three of a Kind | Three cards of the same rank, |
| 2 cards of two other ranks | ||
| 8 | Two Pair | Two cards of the same rank, |
| two cards of another rank, | ||
| one card of a third rank | ||
| 9 | One Pair | Three cards of the same rank, |
| 3 cards of three other ranks | ||
| 10 | High Card | If no one has any of the above hands, |
| the player with the highest card wins |
___________________________________________________________________________
Counting Poker Hands
Straight Flush
Counting from A-K-Q-J-10, K-Q-J-10-9, Q-J-10-9-8, …, 6-5-4-3-2 to 5-4-3-2-A, there are 10 hands that are in sequence in a given suit. So there are 40 straight flush hands all together.
Four of a Kind
There is only one way to have a four of a kind for a given rank. The fifth card can be any one of the remaining 48 cards. Thus there are 48 possibilities of a four of a kind in one rank. Thus there are 13 x 48 = 624 many four of a kind in total.
Full House
Let's fix two ranks, say 2 and 8. How many ways can we have three of 2 and two of 8? We are choosing 3 cards out of the four 2's and choosing 2 cards out of the four 8's. That would be = 4 x 6 = 24. But the two ranks can be other ranks too. How many ways can we pick two ranks out of 13? That would be 13 x 12 = 156. So the total number of possibilities for Full House is
Note that the multiplication principle is at work here. When we pick two ranks, the number of ways is 13 x 12 = 156. Why did we not use = 78?
Flush
There are = 1,287 possible hands with all cards in the same suit. Recall that there are only 10 straight flush on a given suit. Thus of all the 5-card hands with all cards in a given suit, there are 1,287-10 = 1,277 hands that are not straight flush. Thus the total number of flush hands is 4 x 1277 = 5,108.
Straight
There are 10 five-consecutive sequences in 13 cards (as shown in the explanation for straight flush in this section). In each such sequence, there are 4 choices for each card (one for each suit). Thus the number of 5-card hands with 5 cards in sequence is . Then we need to subtract the number of straight flushes (40) from this number. Thus the number of straight is 10240 – 10 = 10,200.
Three of a Kind
There are 13 ranks (from A, K, …, to 2). We choose one of them to have 3 cards in that rank and two other ranks to have one card in each of those ranks. The following derivation reflects all the choosing in this process.
Two Pair and One Pair
These two are left as exercises.
High Card
The count is the complement that makes up 2,598,960.
The following table gives the counts of all the poker hands. The probability is the fraction of the 2,598,960 hands that meet the requirement of the type of hands in question. Note that royal flush is not listed. This is because it is included in the count for straight flush. Royal flush is omitted so that he counts add up to 2,598,960.
Probabilities of Poker Hands
| Poker Hand | Count | Probability | |
|---|---|---|---|
| 2 | Straight Flush | 40 | 0.0000154 |
| 3 | Four of a Kind | 624 | 0.0002401 |
| 4 | Full House | 3,744 | 0.0014406 |
| 5 | Flush | 5,108 | 0.0019654 |
| 6 | Straight | 10,200 | 0.0039246 |
| 7 | Three of a Kind | 54,912 | 0.0211285 |
| 8 | Two Pair | 123,552 | 0.0475390 |
| 9 | One Pair | 1,098,240 | 0.4225690 |
| 10 | High Card | 1,302,540 | 0.5011774 |
| Total | 2,598,960 | 1.0000000 |
___________________________________________________________________________
2017 – Dan Ma
In last week's article, we looked at the basic components of EV calculations. We made two very important points that we're going to use this week:
- The EV of an event is equal to the EVs of each possible outcome added together.
- The EV of an individual outcome is the chance it happens multiplied by the profit when it happens.
We also broke down how to find the EV of any event into a three-step process. If you aren't familiar with that process, then go back and read last week's article because that's the process that we're going to use to evaluate the expected values of some poker situations this week.
For the time being, we aren't going to be concerned with factoring in the rake for these calculations. Once you learn how to do these calculations on a basic level, it's easy to compensate for the rake, and we'll do just that once we get into more in-depth problems.
A Basic Bluffing Scenario
Suppose we're on the river with the nut low against a single opponent who has us covered. The pot is $15, and we make a bet of $10. If our opponent folds, then we win the hand. If our opponent does anything besides fold, then we lose. What is the EV of our bluff if we estimate that our opponent will fold 35 percent of the time?
The first thing we need to do is identify all of the possible outcomes. In this scenario, there are really only two possible outcomes. Our opponent can fold, or our opponent will not fold. This gives us the following EV equation for the value of our bluff:
EV of our bluff = EV of opponent folding + EV of opponent not folding
Now we need to find the EV of the outcome of our opponent folding. To do this, we need to know our profit when our opponent folds and the chance of our opponent folding. We will profit the $15 pot when our opponent mucks his hand, and it will happen 35 percent of the time. We multiply these values together to get the EV of the outcome.
EV of opponent folding = $15 * 0.35 = $5.25
Next, we have to find the EV of our opponent not folding. This will happen 65 percent of the time, and our profit will be a loss of $10 since we lose our bet.
EV of opponent not folding = -$10 * 0.65 = -$6.50
Finally, we add the EV of each outcome together to get our overall EV of our bluff.
EV of our bluff = EV of opponent folding + EV of opponent not folding
EV of our bluff = $5.25 + (-$6.50) = -$1.25
So on average, we will lose $1.25 each time that we make this bluff.
A Basic Calling Scenario
In a $0.05/0.10 game with $2.25 stacks before the blinds are posted, it folds to the small blind who goes all-in. We're in the big blind and decide to call. If we have 55 percent equity against our opponent's range, then what is the EV of our call?
First thing's first: We have to figure out all of the possible outcomes. For our purposes, we can say that we'll either win or lose. That gives us the following EV equation:
EV of calling = EV of winning + EV of losing
Like before, we have to find the EV of each of these outcomes to find the overall EV of calling. The chance of winning is 55 percent since that's our equity, and we'll profit $2.35 because we'll get our opponent's entire starting stack plus our $0.10 blind that was posted. That makes the EV of the winning outcome the following:
EV of winning = $2.35 * 0.55 = $1.29
Now let's find the EV of losing. We know that we'll lose 45 percent of the time because we have 55 percent equity. When we call, we'll actually be calling $2.15 since our starting stack was $2.25 and we posted a $0.10 blind. That means that if we lose, we're losing $2.15.
Poker Scenarios
EV of losing = -$2.15 * 0.45 = -$0.97
Online poker freerolls 2020 results. Like always, we'll add together the EVs of each individual outcome to get the total overall EV of the betting option.
EV of calling = EV of winning + EV of losing
EV of calling = $1.29 + (-$0.97) = $0.32
Pirate's Rose Xtra Reward Slot Machine Bonus - Free Spins BIG WIN. BonusSlotMaster. Playing the Pirate's Rose slot machine, one of Konami's Xtra Reward Slots that have additional features, such as wild reels and random bonuses. Xtra reward slots online casino. Earn XTRA savings on your regular fuel spends with IndianOil XTRAREWARDS Loyalty Program, India's largest and most trusted fuel network.
From our quick calculation, we see that we'll be making about $0.32 each time we make this call, on average.
The Principles in Action
What we want to see from these two examples is that if we follow the basic principles that we have outlined so far, then the calculations themselves are not very difficult at all. This approach is great for people who aren't particularly comfortable with math because it gives them a very straight-forward way of doing EV calculations for basic scenarios without having to really learn a lot of algebra or get in over their head when it comes to the math side of things.
The first principle that you have to know is that the EV of an event or betting action is found by adding up each individual EV of each of the possible outcomes. While both of our examples this week only had two possible outcomes, we're going to look at some more complicated scenarios next week that have three or more.
The second principle that you have to learn is that the EV of an outcome is found by multiplying the profit of that outcome with the chance of that outcome happening. As you can see in the examples above, your profit can be positive or negative, and the chance of an outcome happening can be found with either a fraction or a percentage.
Next week, we're very specifically going to look at semi-bluffing against a single opponent and pure bluffing against multiple opponents. These are two very good situations for learning a lot about the principles of poker with a very minimal amount of math. Meinmmo casino heist.
Poker Scenarios Who Wins
One More Thing
Delegation Poker Scenarios
I just want to point out that FTR has a chat room that's easy to access. I'm in there pretty often, so click here and follow the easy instructions to come chat about poker with us. We don't care if you're a complete beginner or a seasoned veteran — we would love to have you either way!

